Since my walk to 4th place at the Suriname Marathon, I have wanted redemption and to run a fast marathon. The thing is that there aren't too many marathons(despite what you may think from Guyana and Suriname having back to back marathons a week a part) in this neck of the woods. However, after a web search, I did find one in Trinidad in mid- January. Luckily, flights to Trinidad are super cheap and I was actually able to change my flight to include Trinidad with my Barbados trip( I am meeting Kenny in Barbados after the race) for a minimal price change. So I'm doing another marathon in about a month....
Walking those last 6 miles in Suriname is still vivid in my mind and I do not want to do that again. So I've been ramping up my mileage. The thing is, I do a fair amount of trails so I have had to guess how far I've been going. I've got a rough estimate of how far I've gone but it's pretty much just based on my estimate of distance based on how long it took me. Which can be variable with my having to dodge cow droppings and jump trenches.
An easier way to do it would be to use some math. I can use the pythagorean theorem to do this.
c
=
a
2
+
b
2
For example, one trail is the hypotenuse of two roads whose mileage I do know. While it may not be an exact 90 degree triangle it's pretty close. So the sides are: 1.2km and 1.3km. So 1.2 squared plus 1.3 squared equals my distance squared(so I'll need to take the square root of it). So it's about 1.77 km or slightly over a mile. Not bad. Definitely a closer estimate to the actual distance.
If you've ever run a race outside of the US, you've probably noticed that the mile markers come a lot sooner. Like every kilometer or so. Oh! That's probably because they're kilometer markers! Stupid logical measurement system. So if you're like me you probably only think in miles(sorry all you European readers). And will most of us know the basics like: 8 kilometers=5 miles, 13 kilometers= 8 miles, 21 kilometers=13 miles if you delve into the recesses of your mind, you may remember Fibonacci's principle which is an approximation of the Golden spiral or ratio. The golden ratio, not to get too mathy on you, is when two lengths have a ratio(the longer one over the shorter one) that's equal to the sum of the two over the longer one. Meaning: if you divided larger over the smaller you'll get a number that's the same if you add the two and then divide it over the larger number. To steal from wikipedia the equation looks like this:
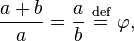
So someone discovered that that ratio is 1.61... and then Fibonacci came up with a sequence of numbers that approximated the Golden ratio. And they are 1, 2, 3, 5, 8, 13, 21, 34, 55, 89 ... ad infinitum. The cool thing is, while the above math is pretty complex-at least for my simple mind, Fibonacci's principle is pretty simple to figure out. You start with 1,2 and then just add them together and get the next number. So 1+2=3, 3+2=5, 5+3=8 etc.
What's even cooler is that this pattern is seen everywhere. Music has it is as the basis for its musical notes. In art, Da Vinci, amongst others, used it. It's even seen in nature. Actually it's seen in nature the most. From trees to galaxies to sea shells.
It is amazing that this sequence, or ratio, is found in so many places in our lives. It doesn't surprise me that running is one of them. Patterns and consistency is a foundation of running and training(just look at the data collection most runners put when writing in their training logs) and this is just another way to express how beautiful running really is:) And another fun example of the intersections of math and running.
Until next time,
Danny
I did not undestand one thing in this blog lol ! Be safe Dan !
ReplyDelete